Delving into the Fundamental Concepts
Internal energy is a crucial thermodynamic property that quantifies the total energy contained within a system. It encompasses the energy associated with various forms, including molecular motion, chemical bonds, and intermolecular forces. Understanding how to calculate the change in internal energy provides invaluable insights into the behavior of systems and their interactions with the surroundings.

Image: www.homeworklib.com
In this comprehensive guide, we will delve into the intricacies of calculating internal energy and its change. We will explore the historical roots, fundamental principles, and practical applications, equipping you with the knowledge to navigate the fascinating world of thermodynamics.
The Origins of Internal Energy
The concept of internal energy emerged during the 19th century as scientists sought to explain the relationship between heat and work. Early experiments by Joule and Mayer established the mechanical equivalent of heat, demonstrating the interconversion between mechanical energy and thermal energy.
Defining Internal Energy and Its Change
Internal energy (U) is a state function that depends solely on the current state of a system, irrespective of the path taken to reach that state. The change in internal energy (ΔU) is the difference in internal energy between two states of a system. It represents the net energy transferred into or out of the system, excluding any work done by the system or on the system.
Calculating Change in Internal Energy
The change in internal energy can be calculated using various methods, depending on the process undergone by the system. Here, we present two primary methods:
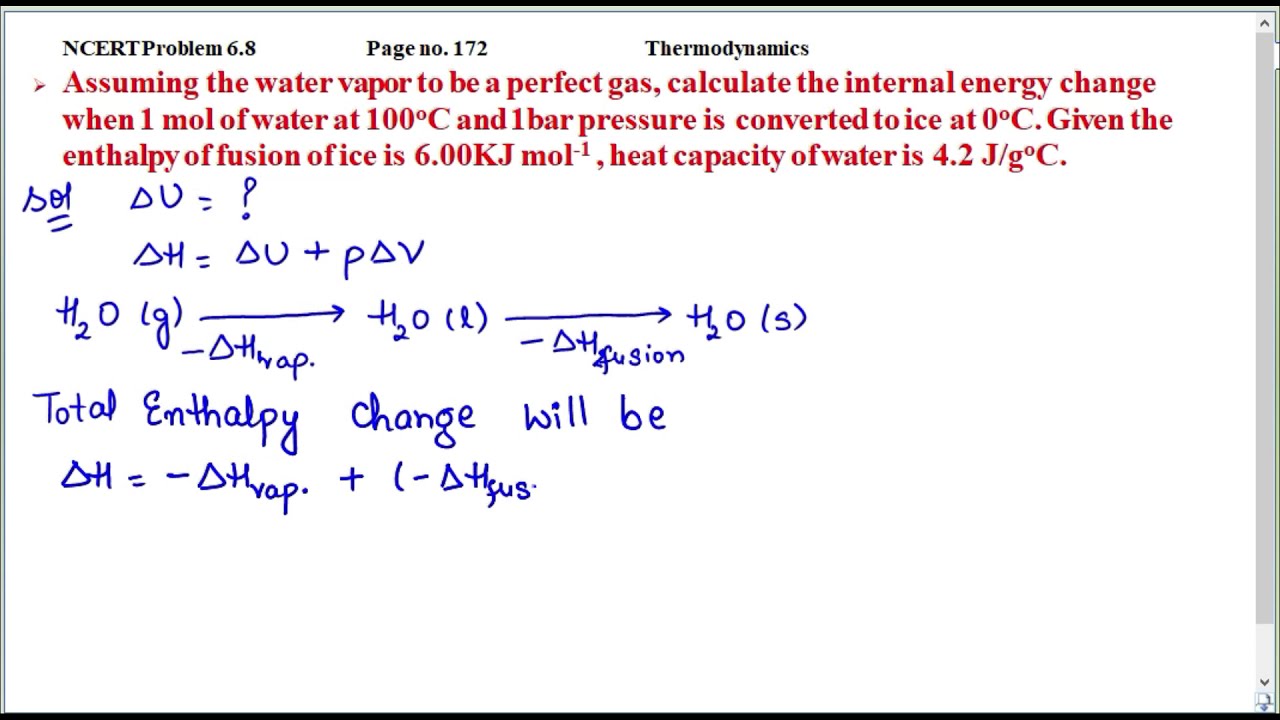
Image: www.vrogue.co
1. Isochoric Process (Constant Volume)
For an isochoric process, where the volume remains constant, the change in internal energy is equal to the heat transferred to the system:
ΔU = Q
2. Isobaric Process (Constant Pressure)
For an isobaric process, where the pressure remains constant, the change in internal energy is given by:
ΔU = Q – PΔV
Here, Q represents the heat transferred, P is the constant pressure, and ΔV is the change in volume of the system.
Applications of Internal Energy Calculations
Calculating and understanding the change in internal energy finds numerous applications across diverse fields:
1. Chemical Reactions
The change in internal energy provides insights into the enthalpy changes associated with chemical reactions, enabling the prediction and optimization of reaction pathways.
2. Phase Transitions
Internal energy changes accompany phase transitions such as melting, freezing, and vaporization. These calculations help determine the energy required to induce these changes.
3. Thermal Expansion
The change in internal energy can be used to predict the thermal expansion of solids, liquids, and gases, facilitating the design of materials with specific thermal properties.
4. Heat Engines and Refrigerators
Internal energy calculations are crucial for analyzing the efficiency and performance of heat engines and refrigerators, devices that convert heat into work or cool a system below the ambient temperature.
How To Calculate Change In Internal Energy
Conclusion
Calculating the change in internal energy offers a powerful tool for understanding the behavior of systems and their interactions with their surroundings. By comprehending the fundamental principles and diverse applications of internal energy, scientists, engineers, and researchers gain invaluable insights into the field of thermodynamics. This knowledge empowers them to predict and control systems, revolutionizing technologies and advancing our understanding of the physical world.